LHC EW precision sub-group DY resummation benchmark
(see also General Meeting last February)
$$ \log(m_Z/q_T)$$
Improved power counting $$ \log(m_Z/q_T) \sim 1/\alpha_s $$
$$ \alpha_s \left(\alpha_s \log\left(\frac{m_Z}{q_T}\right)\right)^n$$ e.g. is the new $\alpha_s$ (NNLL)
Different benchmark levels
$Z$ at 13 TeV, $Q=m_Z$, $y=0$Level 1 & 2
- only resummation, at different N$^k$LL, no non-perturbative model
(Landau pole regulated similarly)
Level 3
- include matching, default settings for Landau pole; physical spectrum
Uncertainties at level 3
- Resummation: e.g. resummation scale, hard scale, rapidity scale
- Matching: e.g. variation of transition function
- Fixed order: e.g. factorization and renormalization scale
See https://indico.cern.ch/event/1108518/ for a full overview
'21, '22, moving beyond: Fixed-order $\alpha_s^3$ and logarithmic $\alpha_s^3$ accuracy while counting $\log(q_T^2/Q^2) \sim 1/\alpha_s$: N$^4$LL+N$^3$LO up to N$^3$LO PDF's!
- three-loop beam functions M.-x. Luo, T.-Z. Yang, H. X. Zhu, Y. J. Zhu '19, '20; Ebert, Mistlberger, Vita '20
Fixed-order Z+jet NNLO calculation
- via 1-jettiness slicing Boughezal, Focke, Liu, Petriello; Boughezal, Campbell, Ellis, Focke, Giele, Liu, Petriello '15
- via antenna subtractions Gehrmann-De Ridder, Gehrmann, Glover, Huss, Morgan '15
- N$^4$LL: Four loop rapidity anomalous dimensionDuhr, Mistlberger, Vita '22; Moult, H.X. Zhu, Y. J. Zhu '22
- e.g. Four-loop collinear anomalous dimensionAgarwal, von Manteuffel, Panzer, Schabinger '21
- Massive three-loop axial singlet contributions Chen, Czakon, Niggetiedt '22
- Two-loop axial singlet V+jet Gehrmann, Peraro, Tancredi '22
Theory uncertainties
- Fixed-order expansions in QCD and EW
- Higher-order resummation
- Parton showers
- Non-perturbative effects, PDFs, TMDs
- Higher power/twist terms in factorization
- Understanding universality of tuning
- Numerical precision
- ...
Fully theory-driven SM measurement far away from 10 MeV,
even with state-of-the-art results
Resummation at $\alpha_s^3$
- via small-$q_T$ factorization
$$ \mathrm{d}\sigma_{ij} \sim \int \mathrm{d}\xi_1 \mathrm{d}\xi_2\, \mathrm{d}\sigma^0_{ij} \cdot H(\xi_1 p_1, \xi_2 p_2, \mu) \cdot $$
$$ \cdot \int \mathrm{d}^2 x_\perp e^{-iq_\perp x_\perp} (x_T^2 Q^2)^{-F(x_\perp,\mu)} \cdot B_i(\xi_1,x_\perp,\mu) \cdot B_j(\xi_2,x_\perp,\mu) $$
based on formalism of Becher, Neubert '10; Becher, Neubert, Wilhelm '11; Becher, Hager '19
implemented in CuTe-MCFM (Becher, Neumann '19)
- three-loop beam functions M.-x. Luo, T.-Z. Yang, H. X. Zhu, Y. J. Zhu '19, '20; Ebert, Mistlberger, Vita '20
- Z+jet NNLO calculation (via 1-jettiness slicing)Boughezal, Focke, Liu, Petriello; Boughezal, Campbell, Ellis, Focke, Giele, Liu, Petriello '15
- N$^4$LL: Four loop rapidity anomalous dimensionDuhr, Mistlberger, Vita '22; Moult, H.X. Zhu, Y. J. Zhu '22
- e.g. Four-loop collinear anomalous dimensionAgarwal, von Manteuffel, Panzer, Schabinger '21
- Massive three-loop axial singlet contributions Chen, Czakon, Niggetiedt '22
Public and well supported (you tell me)!
mcfm.fnal.gov
“If a theoretical calculation is done, but it can not be used by any experimentalist, does it make a sound?”
See also "Computational Challenges for Multi-loop Collider Phenomenology:
A Snowmass 2021 White Paper"
Febres Cordero, von Manteuffel, Neumann '22
Public and well supported (you tell me)!
mcfm.fnal.gov
“If a theoretical calculation is done, but it can not be used by any experimentalist, does it make a sound?”
“It's just a push to GitHub!”
See also "Computational Challenges for Multi-loop Collider Phenomenology:
A Snowmass 2021 White Paper"
Febres Cordero, von Manteuffel, Neumann '22
RadISH+MATRIX
N$^3$LL+NNLO ($\alpha_s^2$)Kallweit, Re, Rottoli, Wiesemann '20
DYTurbo
N$^3$LL+NNLO ($\alpha_s^2$)Camarda, Cieri, Ferrera '21 but can use external $\alpha_s^3$ fixed-order for N$^4$LL$_p$
N$^4$LL$_p$ + N$^3$LO ($\alpha_s^3$) Neumann, Campbell '22; Becher, Neumann '20allows for NNLO $Z$+jet calculation to be used by other codes (DYTurbo)
MiNNLO-PS+POWHEG
Parton shower + NNLO Monni, Nason, Re, Wiesemann, Zanderighi '20
Fiducial results in comparison with CMS 13 TeV data
CMS measurement: 1909.04133
Fiducial results in comparison with CMS 13 TeV data
Cure for Jacobian peak in lepton $q_T$
Why can we use a 5 GeV cutoff for the N$^4$LL$_p$ matching corrections?
Total fiducial cross-sections
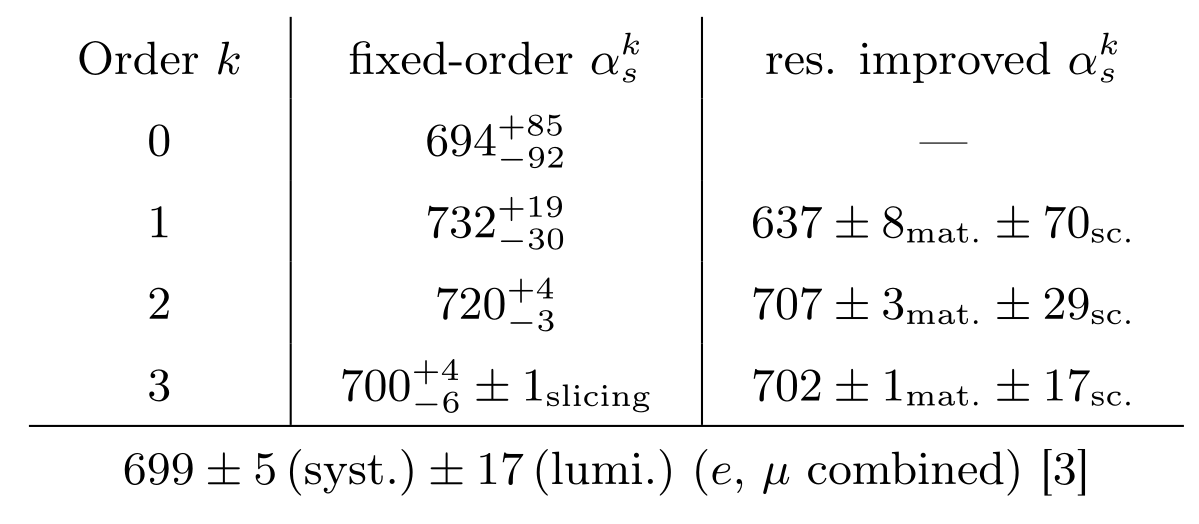
Why can we use a 5 GeV cutoff for N$^3$LO $q_T$ subtractions?
How about for the Tevatron (W-mass)...
This doesn't look too bad...
Non-perturbative effects
In the Becher, Neubert formalism (as implemented in CuTe-MCFM):
Enhanced non-perturbative effects through the collinear anomaly Becher, Bell '13
$$ (b_T^2 Q^2 )^{-F_{ij}(b_T)}, F_{ij} \to F_{ij} + \Lambda_\text{NP,ij}^2 b_T^2 $$
OPE analysis of NP power corrections; $\Lambda_\text{NP,ij}$ has matrix-element definition
Typical $b^*$ prescription is ad-hoc and intertwines perturbative and non-perturbative physics
$$ f(x,b_T) \to f^\text{pert.}(x,b^*(b_T)) F_{b^*}^\text{NP}(b_T) $$ Typical choice $$ F^\text{NP}_{b^*} \sim e^{-\Lambda^2 b_T^2} $$Formalism to include non-perturbative OPE effects model-independently for $b^*$ prescription: Ebert, Michel, Stewart, Sun '22
Preliminary CuTe-MCFM results to appear as Neumann, Campbell '23
Preliminary CuTe-MCFM results to appear as Neumann, Campbell '23
An $\alpha_s^3$ + non-pert. + NLO EW event generator
- Differential grid in Z kinematics $\frac{\mathrm{d}\sigma}{\mathrm{d}y\mathrm{d}q_T\mathrm{d}Q}$ for Z polarisations
- At the level of $\alpha_s^3$ in QCD (N$^3$LO+N$^4$LL$_p$) (+ possibly NLO EW)
- Decay Z using Photos (resummed QED FSR) Golonka, Was '06
- $\to$ Generate events based on generated probability grid
Is there a place for this?
Outlook
- Hadronic SM $W$-mass measurement is complex!
- Decades of work ahead (to squeeze out 10 MeV in less data driven way)
- We need to aim for public and reliable pipelines:
- on both the theory side
- and the experimental analysis side
- Theory: Combine higher-order QCD,EW effects, higher-order resummation, non-perturbative effects into a parton-shower MC
- Allow for higher-order tuning, less guesstimating and to increase universality of tuning